The snub disphenoid
The snub disphenoid or siamese dodecahedron is Johnson solid number 84:

Snub disphenoid
26 pieces: 8 balls, 18 rods (153.40 g)
|
A ring can be made from 14 snub disphenoids and 14 square pyramids:

Ring A of 14 snub disphenoids
392 pieces: 98 balls, 280 rods, 14 squares (2.18 kg)
|
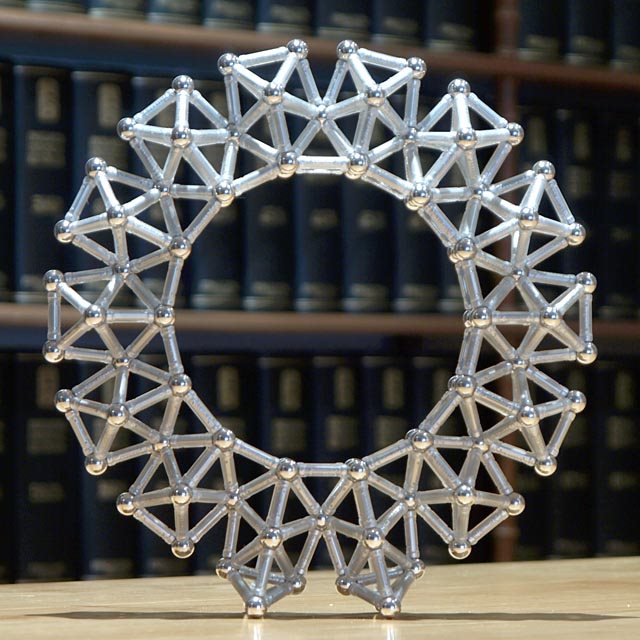
Ring A of 14 snub disphenoids in an upright position
Additional pieces for the feet: 16 pieces: 4 balls, 12 rods
|
The ring, found by Karl Horton, is not exact but it is very nearly so, it has only 0.63 degrees missing. It is also very strong and can be easily placed in an upright position, although the ball which joins each pair of objects receives two rods at too acute an angle, which somewhat weakens that joint.
In the previous ring all 14 objects are placed in a radial orientation. In the following alternative design seven of them are oriented upwards and seven downwards:
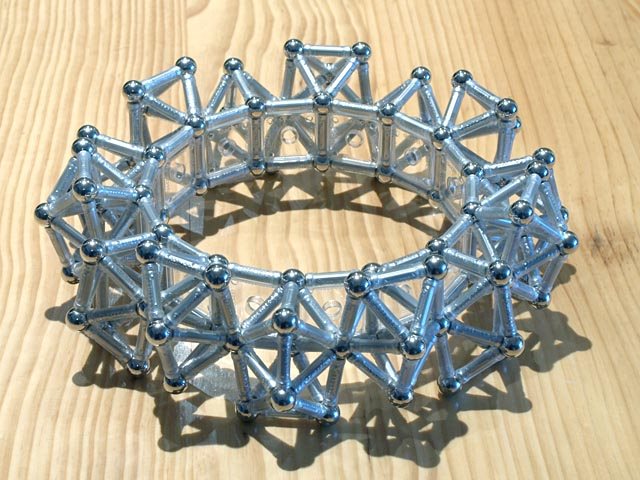
Ring B of 14 snub disphenoids
392 pieces: 98 balls, 280 rods, 14 squares (2.18 kg)
|
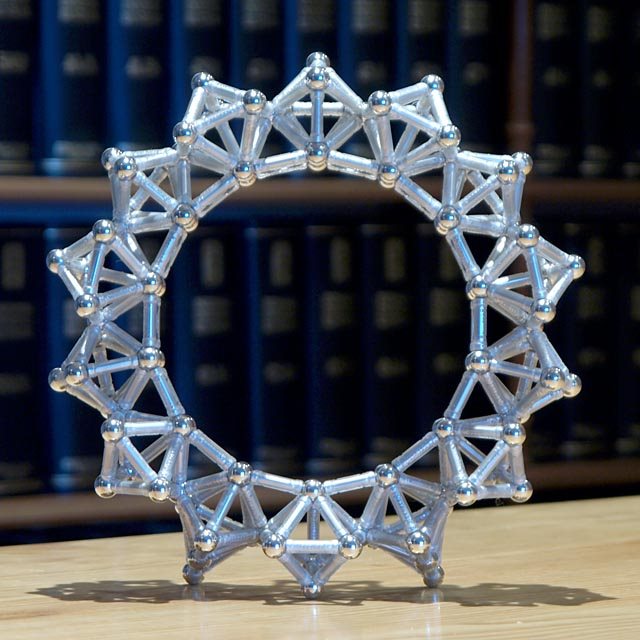
Ring B of 14 snub disphenoids in an upright position
Additional pieces for the feet: 8 pieces: 2 balls, 6 rods
|
This ring is easily stackable:

Stacking of rings B, view 1
3015 pieces: 708 balls, 2195 rods, 112 squares (16.61 kg)
|

Stacking of rings B, view 2
|