The regular octahedron
We have already shown the intimate relationship between the regular octahedron and the regular tetrahedron. We use now 102 octahedra and 192 tetrahedra to build a giant octahedron:
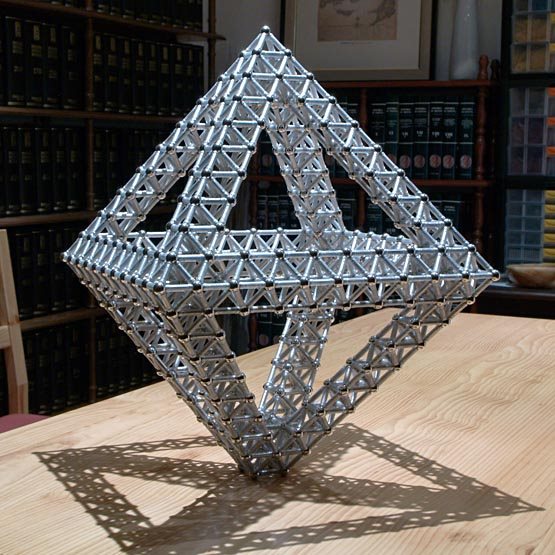
Giant regular octahedron
1656 pieces: 372 balls, 1284 rods (9.23 kg)
|
In the previous picture, the lower ball and the lower four rods (one half octahedron) have been removed in order for the object to be able to stand upright. If complete, it will only be stable when resting on a triangular face:
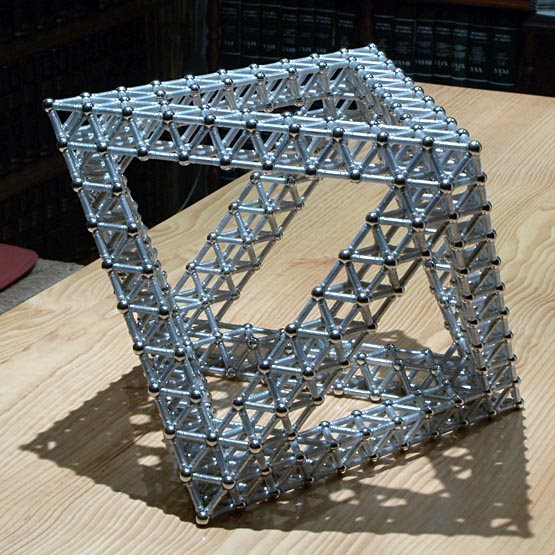
Giant regular octahedron as a triangular antiprism
|
In this position, the octahedron shows its alternative personality as a triangular antiprism, often used for building columns:

Two stacked giant octahedra, view 1
3159 pieces: 693 balls, 2466 rods (17.55 kg)
|

Two stacked giant octahedra, view 2
|
Our giant octahedron is built to scale 10, that is, its edge is made of 10 rods. Using the same construction method, it can be built to other scales beginning in 5. The following table shows the required piece quantities for several scales, and the general formulas:
| scale | balls | rods | |
| 5 | 132 | 504 | |
| 6 | 180 | 660 | |
| 7 | 228 | 816 | |
| 8 | 276 | 972 | |
| 9 | 324 | 1128 | |
| 10 | 372 | 1284 | |
| 11 | 420 | 1440 | |
| 12 | 468 | 1596 | |
| ... | ... | ... | |
| n | 48 × n – 108 | 156 × n – 276 |
- Building Chains of octahedra is easy.