Tetrahedric curves
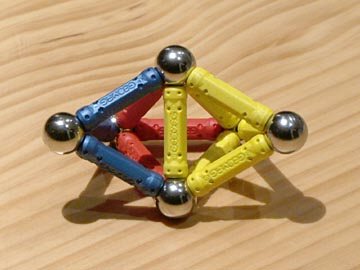
Two regular tetrahedra can be joined by any of their faces. The resultant body is an equilateral triangular hexahedron called the triangular dipyramid in the Johnson classification, which assigns the number 12 to it:
Aggregate of two tetrahedra
|
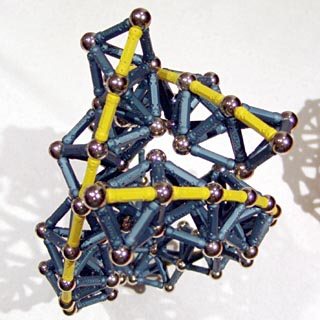
As the six faces of this body are equal, adding a third tetrahedron to any of them results always in the same object:
Aggregate of three tetrahedra, view 1
|
Aggregate of three tetrahedra, view 2
|
We will build several «curves» using this object as a module or building block. First, the following segment, which can be prolonged, uses three modules to make a «curve» which progresses following a straight line, but has three contiguous edges which describe three corresponding helices (blue rods):
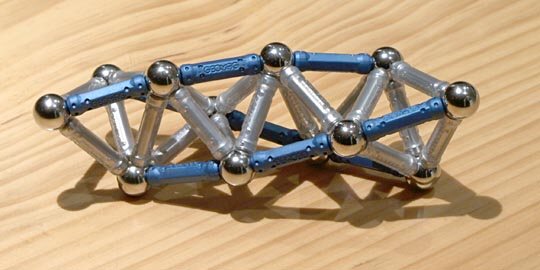
Helical tetrahedric curve segment
|
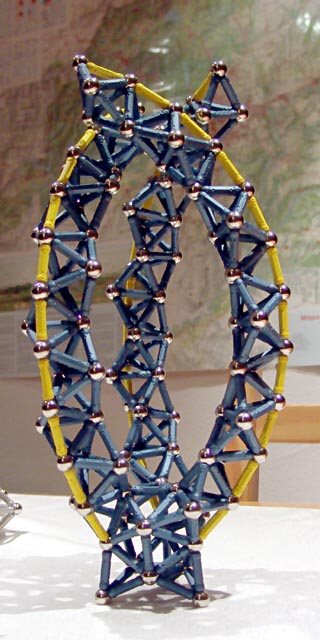
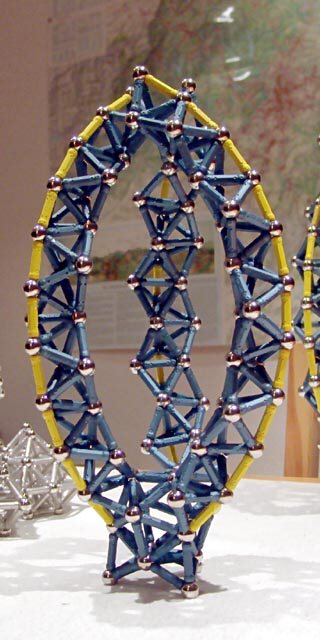
Next, four modules make a segment, also prolongable, having a contiguous edge which describes a circular arc (blue rods):
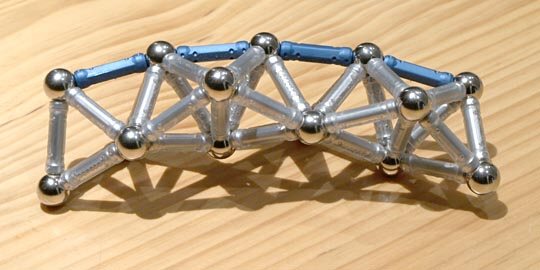
Circular tetrahedric curve segment, view 1
|
Circular tetrahedric curve segment, view 2
|
Promising as they seem, these curves have somewhat frustrating properties. One would expect the circular one to close, forming a full circle, after certain number of repetitions. However, after 32 segments, it just falls a bit short, and cannot be closed.
The helical one, on the other hand, would be expected to repeat the same position, or at least to have a parallel face, after not too many repetitions, but it doesn't. This property would have been very useful in a variety of building situations.
Also they are a bit too weak, and not very fault-tolerant: just a misoriented rod is usually fatal. In any case, the circular one is irreplaceable for certain constructions, as, for instance, the domes of chapels three, four, and five.
Two building examples follow, both using the circular curve. Three segments are built up from a base regular icosahedron which has three tetrahedrical legs. A slightly different position of the base attachments makes the left-hand curves cross in space without touching one another, while the right-hand ones incide in a common vertex:
Three non-incident circular curves, side view
438 pieces: 111 balls, 327 rods (2.49 kg)
 |
Three incident circular curves, side view
388 pieces: 97 balls, 291 rods (2.20 kg)
|
Three non-incident circular curves, top view
|
Three incident circular curves, top view
|
Building these curves to double scale is easy: plainly, the tetrahedra which they are made of must also be built to double scale. A special reinforced icosahedron is required for a base, however. Two views of the incident version to double scale:
Three incident circular curves to double scale, side view 1
1927 pieces: 406 balls, 1521 rods (10.64 kg)
|
Three incident circular curves to double scale, side view 2
|
Common vertex detail:
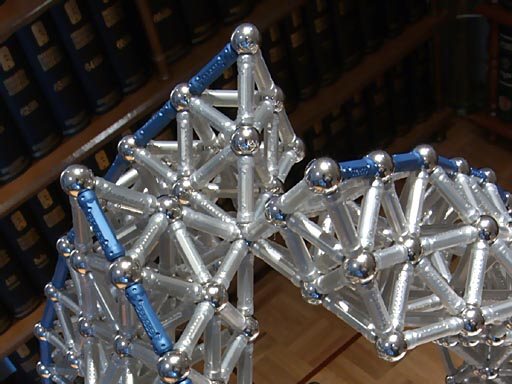
Three incident circular curves to double scale, detail
|
We can even nest the single-scale version inside the double-scale one:
Nested incident circular curves, side view 1
2297 pieces: 497 balls, 1800 rods (12.73 kg)
|
Nested incident circular curves, side view 2
|
